Check back later, or email me if you'd enjoy seeing this work in real-time.
Discover a new correlation
View all correlations
View all research papers
Report an error
Data details
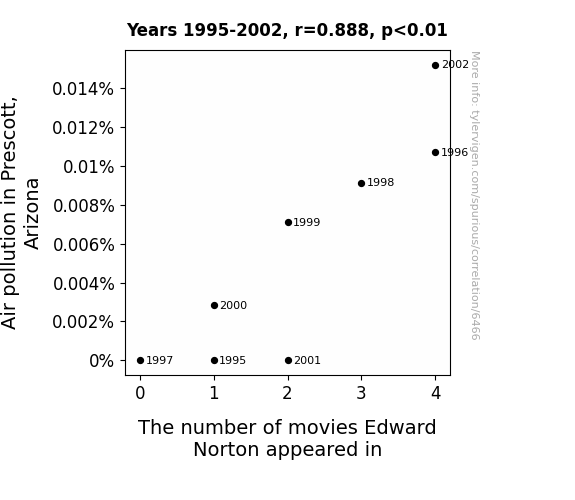
Air pollution in Prescott, ArizonaDetailed data title: Percentage of days 'unhealthy' or worse air quality in Prescott, AZ
Source: Environmental Protection Agency
See what else correlates with Air pollution in Prescott, Arizona
The number of movies Edward Norton appeared in
Source: The Movie DB
Additional Info: American History X (1998); Fight Club (1999); 25th Hour (2002); The Illusionist (2006); Down in the Valley (2005); Red Dragon (2002); Leaves of Grass (2009); Pride and Glory (2008); The Painted Veil (2006); The Apple Pushers (2012); The Incredible Hulk (2008); Motherless Brooklyn (2019); Behind Bars: The Making of Stone (2011); Mysteries of the Jules Rimet Trophy (2014); High Noon on the Waterfront (2022); Between the Devil and the Deep Blue Sea (1995); The 53rd Calypso (1996); Bustin' Down the Door (2008); Rounders (1998); Keeping the Faith (2000); The Score (2001); Death to Smoochy (2002); Everyone Says I Love You (1996); Stone (2010); Collateral Beauty (2016); Glass Onion: A Knives Out Mystery (2022); Comedy Central Roast of Bruce Willis (2018); UnCharitable (2023); Primal Fear (1996); The People vs. Larry Flynt (1996); The Italian Job (2003); The Bourne Legacy (2012); Birdman: All-Access (A View From the Wings) (2015); Source of Pride: The Making of "Pride and Glory" (2009); Paper Birds (2021); The Evolution of an American Filmmaker (2003); Out of the Past (1998); Moonrise Kingdom (2012); Birdman or (The Unexpected Virtue of Ignorance) (2014); Isle of Dogs (2018); Moonrise Kingdom: Welcome to the Island of New Penzance (2012); A Director's Journey: The Making of 'Red Dragon' (2003); Kingdom of Heaven (2005); Starz Inside: Comic Books Unbound (2008); Asteroid City (2023); Exploring the Set of 'Moonrise Kingdom' (2015); The Grand Budapest Hotel (2014); Frida (2002); Declaration of Independence (2003); Brando (2007); Belmondo l'influenceur (2020); The Making of The Grand Budapest Hotel (2014); Sausage Party (2016); Forever Hollywood (1999); The Invention of Lying (2009); Killers Kill, Dead Men Die (2007); The French Dispatch (2021); After the Sunset (2004); The Concert for New York City (2001); Salinger (2013); Alita: Battle Angel (2019); Saturday Night Live: 40th Anniversary Special (2015); The Dictator (2012)
See what else correlates with The number of movies Edward Norton appeared in
Correlation is a measure of how much the variables move together. If it is 0.99, when one goes up the other goes up. If it is 0.02, the connection is very weak or non-existent. If it is -0.99, then when one goes up the other goes down. If it is 1.00, you probably messed up your correlation function.
r2 = 0.7891359 (Coefficient of determination)
This means 78.9% of the change in the one variable (i.e., The number of movies Edward Norton appeared in) is predictable based on the change in the other (i.e., Air pollution in Prescott, Arizona) over the 8 years from 1995 through 2002.
p < 0.01, which is statistically significant(Null hypothesis significance test)
The p-value is 0.0032. 0.0031960375997503654000000000
The p-value is a measure of how probable it is that we would randomly find a result this extreme. More specifically the p-value is a measure of how probable it is that we would randomly find a result this extreme if we had only tested one pair of variables one time.
But I am a p-villain. I absolutely did not test only one pair of variables one time. I correlated hundreds of millions of pairs of variables. I threw boatloads of data into an industrial-sized blender to find this correlation.
Who is going to stop me? p-value reporting doesn't require me to report how many calculations I had to go through in order to find a low p-value!
On average, you will find a correaltion as strong as 0.89 in 0.32% of random cases. Said differently, if you correlated 313 random variables Which I absolutely did.
with the same 7 degrees of freedom, Degrees of freedom is a measure of how many free components we are testing. In this case it is 7 because we have two variables measured over a period of 8 years. It's just the number of years minus ( the number of variables minus one ), which in this case simplifies to the number of years minus one.
you would randomly expect to find a correlation as strong as this one.
[ 0.49, 0.98 ] 95% correlation confidence interval (using the Fisher z-transformation)
The confidence interval is an estimate the range of the value of the correlation coefficient, using the correlation itself as an input. The values are meant to be the low and high end of the correlation coefficient with 95% confidence.
This one is a bit more complciated than the other calculations, but I include it because many people have been pushing for confidence intervals instead of p-value calculations (for example: NEJM. However, if you are dredging data, you can reliably find yourself in the 5%. That's my goal!
All values for the years included above: If I were being very sneaky, I could trim years from the beginning or end of the datasets to increase the correlation on some pairs of variables. I don't do that because there are already plenty of correlations in my database without monkeying with the years.
Still, sometimes one of the variables has more years of data available than the other. This page only shows the overlapping years. To see all the years, click on "See what else correlates with..." link above.
| 1995 | 1996 | 1997 | 1998 | 1999 | 2000 | 2001 | 2002 | |
| Air pollution in Prescott, Arizona (Bad air quality days) | 0 | 0.0106952 | 0 | 0.00913242 | 0.0070922 | 0.00282486 | 0 | 0.0152091 |
| The number of movies Edward Norton appeared in (Movie appearances) | 1 | 4 | 0 | 3 | 2 | 1 | 2 | 4 |
Why this works
- Data dredging: I have 25,153 variables in my database. I compare all these variables against each other to find ones that randomly match up. That's 632,673,409 correlation calculations! This is called “data dredging.” Instead of starting with a hypothesis and testing it, I instead abused the data to see what correlations shake out. It’s a dangerous way to go about analysis, because any sufficiently large dataset will yield strong correlations completely at random.
- Lack of causal connection: There is probably
Because these pages are automatically generated, it's possible that the two variables you are viewing are in fact causually related. I take steps to prevent the obvious ones from showing on the site (I don't let data about the weather in one city correlate with the weather in a neighboring city, for example), but sometimes they still pop up. If they are related, cool! You found a loophole.
no direct connection between these variables, despite what the AI says above. This is exacerbated by the fact that I used "Years" as the base variable. Lots of things happen in a year that are not related to each other! Most studies would use something like "one person" in stead of "one year" to be the "thing" studied. - Observations not independent: For many variables, sequential years are not independent of each other. If a population of people is continuously doing something every day, there is no reason to think they would suddenly change how they are doing that thing on January 1. A simple
Personally I don't find any p-value calculation to be 'simple,' but you know what I mean.
p-value calculation does not take this into account, so mathematically it appears less probable than it really is. - Very low n: There are not many data points included in this analysis. Even if the p-value is high, we should be suspicious of using so few datapoints in a correlation.
Try it yourself
You can calculate the values on this page on your own! Try running the Python code to see the calculation results. Step 1: Download and install Python on your computer.Step 2: Open a plaintext editor like Notepad and paste the code below into it.
Step 3: Save the file as "calculate_correlation.py" in a place you will remember, like your desktop. Copy the file location to your clipboard. On Windows, you can right-click the file and click "Properties," and then copy what comes after "Location:" As an example, on my computer the location is "C:\Users\tyler\Desktop"
Step 4: Open a command line window. For example, by pressing start and typing "cmd" and them pressing enter.
Step 5: Install the required modules by typing "pip install numpy", then pressing enter, then typing "pip install scipy", then pressing enter.
Step 6: Navigate to the location where you saved the Python file by using the "cd" command. For example, I would type "cd C:\Users\tyler\Desktop" and push enter.
Step 7: Run the Python script by typing "python calculate_correlation.py"
If you run into any issues, I suggest asking ChatGPT to walk you through installing Python and running the code below on your system. Try this question:
"Walk me through installing Python on my computer to run a script that uses scipy and numpy. Go step-by-step and ask me to confirm before moving on. Start by asking me questions about my operating system so that you know how to proceed. Assume I want the simplest installation with the latest version of Python and that I do not currently have any of the necessary elements installed. Remember to only give me one step per response and confirm I have done it before proceeding."
# These modules make it easier to perform the calculation
import numpy as np
from scipy import stats
# We'll define a function that we can call to return the correlation calculations
def calculate_correlation(array1, array2):
# Calculate Pearson correlation coefficient and p-value
correlation, p_value = stats.pearsonr(array1, array2)
# Calculate R-squared as the square of the correlation coefficient
r_squared = correlation**2
return correlation, r_squared, p_value
# These are the arrays for the variables shown on this page, but you can modify them to be any two sets of numbers
array_1 = np.array([0,0.0106952,0,0.00913242,0.0070922,0.00282486,0,0.0152091,])
array_2 = np.array([1,4,0,3,2,1,2,4,])
array_1_name = "Air pollution in Prescott, Arizona"
array_2_name = "The number of movies Edward Norton appeared in"
# Perform the calculation
print(f"Calculating the correlation between {array_1_name} and {array_2_name}...")
correlation, r_squared, p_value = calculate_correlation(array_1, array_2)
# Print the results
print("Correlation Coefficient:", correlation)
print("R-squared:", r_squared)
print("P-value:", p_value)Reuseable content
You may re-use the images on this page for any purpose, even commercial purposes, without asking for permission. The only requirement is that you attribute Tyler Vigen. Attribution can take many different forms. If you leave the "tylervigen.com" link in the image, that satisfies it just fine. If you remove it and move it to a footnote, that's fine too. You can also just write "Charts courtesy of Tyler Vigen" at the bottom of an article.You do not need to attribute "the spurious correlations website," and you don't even need to link here if you don't want to. I don't gain anything from pageviews. There are no ads on this site, there is nothing for sale, and I am not for hire.
For the record, I am just one person. Tyler Vigen, he/him/his. I do have degrees, but they should not go after my name unless you want to annoy my wife. If that is your goal, then go ahead and cite me as "Tyler Vigen, A.A. A.A.S. B.A. J.D." Otherwise it is just "Tyler Vigen."
When spoken, my last name is pronounced "vegan," like I don't eat meat.
Full license details.
For more on re-use permissions, or to get a signed release form, see tylervigen.com/permission.
Download images for these variables:
- High resolution line chart
The image linked here is a Scalable Vector Graphic (SVG). It is the highest resolution that is possible to achieve. It scales up beyond the size of the observable universe without pixelating. You do not need to email me asking if I have a higher resolution image. I do not. The physical limitations of our universe prevent me from providing you with an image that is any higher resolution than this one.
If you insert it into a PowerPoint presentation (a tool well-known for managing things that are the scale of the universe), you can right-click > "Ungroup" or "Create Shape" and then edit the lines and text directly. You can also change the colors this way.
Alternatively you can use a tool like Inkscape. - High resolution line chart, optimized for mobile
- Alternative high resolution line chart
- Scatterplot
- Portable line chart (png)
- Portable line chart (png), optimized for mobile
- Line chart for only Air pollution in Prescott, Arizona
- Line chart for only The number of movies Edward Norton appeared in
Thanks for shining a light on this correlation!
Correlation ID: 6466 · Black Variable ID: 21579 · Red Variable ID: 26508


